Optical microscopes have long been essential tools in many scientific disciplines, particularly the biological and medical sciences. Conventional widefield microscopes—encompassing transmission, phase contrast and fluorescence imaging modes—are the workhorses of many laboratories. Over the last 25 years, researchers have also made significant developments in 3-D imaging using scanning laser microscopes. This progress started with the confocal microscope, which provides 3-D resolution by using a pinhole to exclude out-of-focus light. Rather than produce a whole image simultaneously, these microscopes scan a laser spot through the specimen, building the image point-by-point.
This achievement was followed by several other laser-scanning methods, including the commonly used two-photon fluorescence microscope. Rather than using a pinhole to generate 3-D discrimination, this microscope relies on the nonlinear process of two-photon excitation to ensure that fluorescence is only generated in the focus, where the laser intensity is highest. Various advances in this field have led to improvements in resolution and contrast. Standard laboratory microscopes now regularly produce images revealing 3-D structure on the submicrometer scale. Fluorescence markers show functional information about cellular processes, and Raman-based methods provide chemical specificity. Several new methods of nanoscopy that combine optical and photophysical phenomena can even beat the diffraction limit to resolve details on the tens-of-nanometers scale.
Widefield imaging and laser scanning microscope operation. (Left) In a widefield microscope, a light source illuminates an area of the specimen, which is imaged via the objective lens onto a camera. (Right) In a scanning microscope, a laser is focused by the objective to create a spot in the specimen. The image is built in a point-by-point manner by scanning the spot relative to the specimen.
These methods all rely on careful engineering to ensure that the optics operate at the diffraction limit, so that optimum resolution and efficiency are achieved. However, one part of the optical system—the specimen—lies outside the design specification. It is optically inhomogeneous and exhibits spatially varying refractive indices. Hence, the light focused into the specimen suffers from wavefront distortions—or phase aberrations—that degrade the resolution and imaging efficiency of the microscope. The aberrations vary from one specimen to another, so they cannot be corrected by a fixed optical design. Dynamic correction is necessary. This is where adaptive optics (AO) comes into play.
Adaptive optics was originally conceived for use in astronomical telescopes. These AO systems detect aberrations introduced by the atmosphere and use a deformable mirror to remove the aberrations before the light reaches the imaging detector. For imaging systems with small apertures, such as our eyes, the turbulence causes twinkling; for wider telescope apertures, it leads to severe image blurring that limits the resolution of the telescope.
The AO approach has been widely applied in astronomy, and it has also found application in ophthalmic imaging, laser-based fabrication, optical communications and, of course, microscopy. The adoption of AO for microscopes has brought new challenges that have required innovative solutions.
Aberrations in microscopes
There are two potential sources of aberrations in microscopes: the optics and the specimen. The optical systems of high-resolution microscopes are designed to operate near the diffraction limit, but design compromises are inevitable; thus, there will always be some aberrations. Even high-end microscopes can benefit from further aberration correction.
Introducing the specimen into the system can also compromise even the best optical design. Most biological specimens are 3-D. Advances in microscope technology have enabled volumetric imaging that provides rich information about biological structure and function. The same 3-D structures that are of interest to the microscopist are accompanied by spatial variations in refractive index.
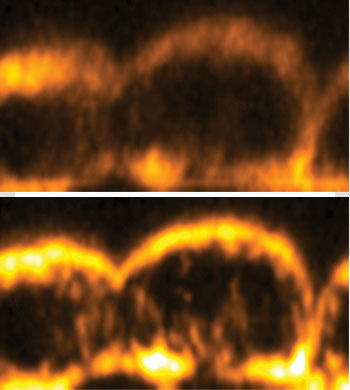Mouse embryo images from an adaptive third harmonic generation microscope. (Top) Without aberration correction. (Bottom) With aberration correction. Aberration correction leads to increased signal and improved resolution, particularly along the optic axis (vertical).
During image acquisition, light in different parts of the focused beam passes through spatial variations in refractive index—which consequently introduces aberrations. This occurs in both the illumination and detection paths. However, depending upon the type of microscope, the aberrations in either of these paths may not affect image quality. For example, both paths are affected in the confocal microscope. Aberrations degrade the intensity and shape of the focused laser spot, but they also affect the imaging of the generated fluorescence onto the pinhole. Conversely, in the two-photon microscope, only aberrations in the illumination path are relevant, as the image resolution is determined solely by the quality of the focal spot.
The nature of the induced aberrations can vary considerably from specimen to specimen. The magnitude and complexity of aberrations increase with the focusing depth, as the effect of the refractive index variations accumulates. For moderate focusing depths, the wavefront distortions are relatively smooth. At larger depths, the more complex distortions give rise to scattering, where both phase and amplitude effects are present.
The properties of specimen-induced aberrations contrast with those experienced by astronomical AO systems. The aberrations caused by atmospheric turbulence are dynamic and require fast adaptation of the aberration correction in order to maintain image quality.
Microscopic specimen-induced aberrations, on the other hand, arise from the sample structure. As specimens are usually stationary over the imaging timescale, the aberrations also remain static. This is the case even when one is observing dynamic cellular processes, since the movements of small cellular subcomponents near the focus have little effect on aberrations compared to the larger bulk structure of the specimen lying above the focus. Although the aberrations are temporally static, they can vary spatially. This could lead to the need for rapid dynamic aberration correction, for example, if one needed to adapt the correction as the laser focus was scanned across the specimen.
The detrimental effects of aberrations on image quality include reduced resolution and a decrease in image brightness and/or contrast. These effects are readily seen as one focuses deeper than a few tens of micrometers into an aberrating specimen. Although increasing the illumination power can often restore image brightness, it has no effect on either resolution or contrast. With high laser intensity, one might acquire an image of sorts at depth, but it is likely to be washed out or dominated by noise. By correcting the aberrations adaptively, one can restore resolution and contrast at each depth in the specimen, and the consequent decrease in illumination power increases the durability of the specimen.
Methods of aberration correction. (Left) A potential applied between the membrane and an electrode exerts a force that deforms the membrane. Aberrations are introduced as phase delays due to the varying optical path length. (Right) The local orientation of the liquid crystal (LC) molecules depends on the applied pixel voltage. As the effective refractive index of the LC molecules changes, the optical path length through each pixel can be modulated to introduce or correct aberrations.
Adaptive optics
The role of an AO system is to dynamically measure and correct aberrations. A conventional AO system, such as that used in an astronomical telescope, consists of a wavefront sensor, a wavefront corrector and a control system. When rightly set, the wavefront corrector introduces a conjugate aberration that cancels out the distortion in the input beam. This correction aberration is updated as the input changes. While the principle of AO is the same in microscopes, the actual implementation has required several other approaches for addressing the challenges encountered in a microscope system.
Two types of wavefront correctors have been used in adaptive microscopes: deformable mirrors and liquid crystal spatial light modulators. A deformable mirror consists of a flexible reflective surface, controlled by a collection of actuators, which may be electrostatic, piezo-electric or electromagnetic. Light reflected off the deformable mirror acquires an additional phase aberration, proportional to the extra optical path length that depends upon the mirror shape. These mirrors produce continuous smooth shapes and have the advantage of wavelength- and polarization-independent operation—properties that make them attractive in both astronomy and microscopy.
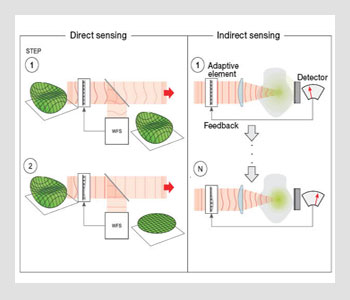Direct vs. indirect sensing. (Left) In direct sensing, an aberrated input wavefront is measured with an AO direct wavefront sensor, which gives the information needed to set the deformable mirror with a conjugate aberration. In closed-loop operation, the mirror is adapted as the input aberration changes. (Right) With indirect sensing, wavefront measurement is conducted with a sensorless AO system. Measurements are taken with predetermined aberrations introduced by the deformable mirror. The aberration correction is then estimated using an appropriate algorithm, optimizing the signal and resolution.
Spatial light modulators (SLMs) are pixelated liquid crystal devices that modulate optical phase through a change in the state of the liquid crystal. These polarization-dependent and chromatically dependent devices are best suited to conditioning monochromatic laser illumination; they are of limited use in correcting fluorescence emission. SLMs have been usefully applied to two-photon microscopes, where correction is only required in the illumination beam. Although SLMs have a limited modulation range—typically up to one wavelength of retardation—they can effectively simulate larger aberrations using phase wrapping, where larger phases are wrapped into the range 0 to 2π radians. SLMs also provide the added flexibility of using phase holograms to manipulate the focal field, for example, to create an array of multiple foci.
Astronomical AO systems use guide stars for wavefront sensing—either bright stars near the objects of interest or artificial beacons created by a laser beam projected into the atmosphere. These guide stars act as a source of wavefronts whose aberrations are measured by a wavefront sensor (such as a Hartmann-Shack sensor or interferometer). In laser scanning microscopes, the focal spot itself can serve as a guide star, but the consequent aberration measurement is less straightforward.
Unlike the point-like guide star source in the telescope, the microscope specimen is full of emitters, in the form of scatterers or fluorophores spread throughout the volume. The light reaching the wavefront sensor emanates not only from the focus, but also from anywhere within the focus cone of the laser. Light can also be scattered between the focus and the sensor, introducing additional phase shifts due to the different optical paths.
In order to measure the aberrations directly and unambiguously, one must exclude out-of-focus light. This can be achieved by using a pinhole to filter out the wavefronts coming from the focal region. A similar principle is used in the confocal microscope, although a larger pinhole aperture would normally be used to avoid filtering out the aberration phase information. A different method uses the coherence properties of light to select light scattered from a limited range of depths using coherence gating.
Another design challenge with AO microscopes is that they are dual-pass systems, where light both enters the specimen for illumination and then propagates back out again in order to be detected. This contrasts with the telescope, in which light passes in a single direction from the star to the detector. The dual-pass nature of the microscope can lead to ambiguous aberration measurements.
Consider the situation in which the illuminating laser beam reflects from a locally flat (mirror-like) interface in the specimen and the back-scattered light is used for the measurement process. A ray passing through one side of the objective lens pupil impinges on the mirror at an angle θ and is reflected at an angle ‒θ. It then passes back through the pupil on the opposite side. The beam is thus inverted about the optical axis. Any aberrations induced on the illumination path are spatially inverted on the reflection path. As a consequence, any odd aberrations (such as coma) are cancelled out, whereas even aberrations (astigmatism, spherical) are doubled in magnitude.
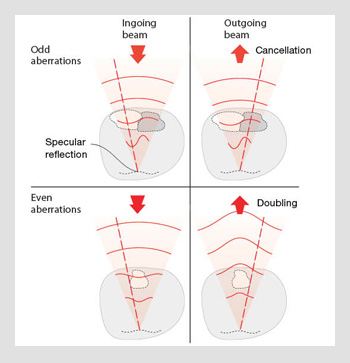Double pass effect. Light is reflected from a mirror-like part of the specimen, leading to the cancellation of odd-symmetry aberrations and the doubling in amplitude of even-symmetry aberrations.
On the other hand, scattering from a point-like object would lead to the correct registration of aberrations by the sensor. This problem can be somewhat alleviated when one uses incoherent fluorescence guide stars, given that the phase of the illumination light is lost in the fluorescence process. Several research groups have been investigating the use of Hartmann-Shack sensors in microscopes, and progress is being made towards their wider use.
The phenomena we describe here create even greater challenges when one is using wavefront sensors in microscopes. For this reason, most AO microscopy uses indirect or “sensorless” methods of aberration measurement. In these systems, the wavefront compensation is determined from a sequence of images, each of which contains an additional aberration that has been intentionally introduced using the adaptive element. Encoded within this set of images is sufficient information for determining the optimum correction aberration.
The required number of images depends upon the algorithm used, but efficient schemes have been developed that minimize this number. Consequently, the time taken and the exposure of the specimen are also reduced. This approach allows for measurement of n aberration modes with as few as n + 1 images, typically taking a few seconds to complete the measurement process.
Progress in microscope applications
AO has been demonstrated in a range of microscope modalities, including conventional widefield microscopes as well as laser scanning systems. The most common implementations have involved confocal and two-photon fluorescence microscopy, both of which are widely used methods in biomedical investigations. Due to aberrations, these microscopes suffer from a significant drop in signal and resolution as the focus is moved deeper into the specimen.
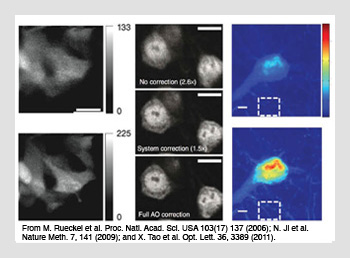Aberration correction in adaptive scanning microscopy. (Left) Coherence gated sensing using two-photon fluorescence of GFP-stained neurons in a developing zebrafish embryo. (Center) Pupil segmentation sensing using two-photon fluorescence of stained neurons under a 300-µm-thick fixed mouse brain slice. (Right) Direct wavefront sensing using confocal fluorescence images of stained neurons under a 100-µm-thick fixed mouse brain slice.
Various research groups have combined these microscopes with direct wavefront sensing and sensorless AO, normally using deformable mirrors for aberration compensation. Ji et al. developed another approach that uses an SLM to implement a pupil segmentation phasing method in a two-photon microscope. AO has also been applied to microscopes using more exotic contrast mechanisms based upon nonlinear optical processes, such as second- and third-harmonic generation or coherent anti-Stokes Raman scattering. Using these various methods, researchers have demonstrated image improvement at depths of up to 100 µm in mouse embryos and over 200 µm in brain tissue.
Adaptive microscopy is also finding a role in the imaging of live specimens. It can help to reduce the time required for image acquisition by increasing signal generation and collection efficiency. This is particularly useful in microscopes that rely on nonlinear contrast mechanisms, where any drop in focal intensity has a compounded effect on signal level.
Related developments
The AO methods we describe are well suited to the compensation of phase aberrations that are relatively smooth in shape. However, other significant problems can be caused in thick tissue microscopy by the effects of scattering. The aberrations manifest themselves as rapid variations in optical phase across the wavefront (also accompanied by variations in amplitude), caused by multiple scattering effects from specimen features.
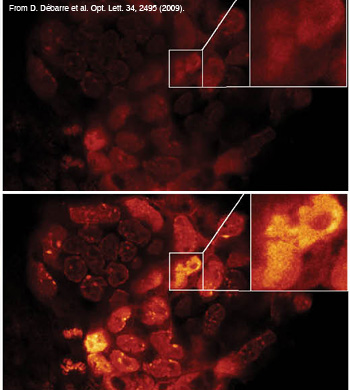Modal image-based AO using two-photon images of a GFP- and DAPI-stained fixed mouse embryo.
One form of AO, first introduced by Vellekoop et al., has been developed to deal specifically with this problem. Like other methods of AO, this approach is based on the principle of phase conjugation. The SLM phase pixels are chosen to maximize the focal intensity. After many optimization measurements, this method can increase focal intensity from a very low level by several orders of magnitude. It does not provide full correction of the phase, in the sense that it cannot achieve the same concentration of illumination power into the focus as the conventional AO regime. It is also limited to narrow band operation, since the scattering effects can change significantly with a shift in wavelength. Although it has yet to be demonstrated in an epi-illumination microscope, this method nevertheless holds great potential for combating the limitations imposed by scattering specimens.
A recent finding from Katz et al. has shown that not only spatial effects of scattering, but also temporal ones, can be compensated—an important result given that nonlinear microscopes usually use short-pulsed femtosecond lasers.
Most optical design involves optimizing a system to ensure that aberrations are kept to a minimum—a challenging task when one has to comply with several sometimes-contradictory requirements. For example, the design of an ideal objective lens for high-resolution microscopy might require high numerical aperture, wide field of view (or equivalently low magnification), low field curvature, high transmission and achromatic operation over more than an octave of wavelengths. This combination may be achievable, but only with extremely complex compound lenses.
AO can help by allowing the designer to relax the aberration tolerance from the usual fraction of a wavelength up to several wavelengths. This permits a significant reduction in the complexity of the optical system. In configurations where the optical fidelity has been compromised, the AO can be used to correct the residual system aberration, restoring diffraction-limited operation. Such a system was developed by Potsaid et al. for increasing the field of view of a microscope. This principle has also been used in the design of harmonic generation microscopes for use at wavelengths where suitable objective lenses were not available.
Future prospects
More work must be done before AO can become a regular component of laboratory microscopes. Most AO microscopes are too complex to set up, and their application can be limited by the robustness of operation. The development of automated alignment and calibration procedures would enable the turnkey operation needed to make these systems more practical.
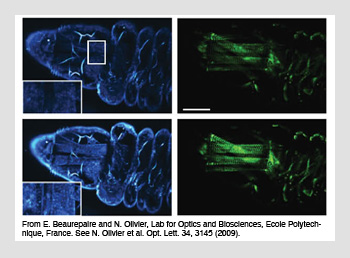Harmonic generation images of a live Drosophila larva (blue, third-harmonic generation and green, second-harmonic generation). The top images of each set are without aberration correction, and the bottom images are after correction.
The effectiveness of AO microscopy is mostly compromised by aberration measurement, rather than by currently available correction devices. More sophisticated wavefront sensors or sensorless optimization schemes will extend the microscope’s ability to cope with large and more complex aberrations. An obvious goal is to develop “real-time” aberration sensing to increase the speed of correction. Coupled to this is the desire to reduce the exposure of specimens during the measurement process—an essential step when using microscopes for live imaging.
Aberrations can change significantly across a single field of view because the refractive index of the specimen varies throughout its volume. So far, the methods used in adaptive microscopes have provided only a fixed aberration correction for each image. This is sufficient if the imaged region is small enough that aberrations do not vary significantly across the field.
One way to overcome this limitation would be to apply multiconjugate AO to microscopes. This method has been applied in astronomy using multiple deformable mirrors to compensate for multiple aberrating layers in the atmosphere. A similar approach in microscopy would compensate for the 3-D refractive index distribution, although the optical system would become considerably more complex.
Further advances in AO will extend the capabilities of high-resolution microscopes to reveal functional and structural information from deep within biological tissue. Currently, optimum performance is often limited to thin regions near to the coverslip, sufficient for imaging individual cells, but of rather limited practicality for tissue imaging. AO promises to help move microscopy into a new regime in which biological studies that were previously confined to cell cultures can be performed in thick tissue and even in live specimens.
Martin Booth is in the department of engineering science at the University of Oxford, U.K. Delphine Débarre is with the Ecole Polytechnique, CNRS, and INSERM, Palaiseau, France. Alexander Jesacher is with Innsbruck Medical University, Austria.
References and Resourcess
>> M.J. Booth. Phil. Trans. R. Soc. A 365, 2829 (2007).
>> J. Girkin et al. Curr. Opin. Biotechnol. 20, 106 (2009).
>> A. Jesacher et al. Opt. Lett. 34, 3154 (2009).
>> O. Katz et al. Nat. Photonics 5, 372 (2011).
>> B. Potsaid et al. Opt. Express 13, 6504 (2005).
>> I. Vellekoop and A. Mosk. Opt. Lett. 32, 2309 (2007).