Optical Engineering
Optomechanics and the Small-Displacement Domain
It’s no secret that many of the analytical methods used by mechanical engineers are linearized simplifications of more or less nonlinear mathematical theories. Structural mechanics and heat transfer are just two of these theories.
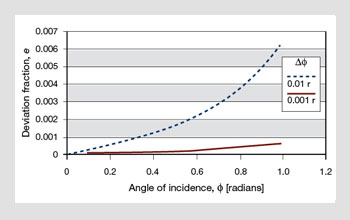
Non-linearity in Snell’s law for n=1.0, n´=1.5
As an optomechanical designer, I have one great advantage over colleagues who perform optical design: They search for solutions in a vast design space that ranges from a tenth of a meter to several tens of meters, while my design space is limited to small structural displacements. Before I even start my design, I know my solutions are on the order of tens of microns or less. It’s true that a direct comparison is not wholly valid, because structural displacements are in the first partial derivative domain with respect to the relatively large optical dimensions. However, this fact also works to the optomechanical designer’s advantage.
As a mechanical designer who has served the optical engineering industries over the past 35 years, I have found it very helpful to be able to imbed “optical figures of merit” in my mechanical engineering tools. Those figures—for imaging systems, interferometers, telecom components and remote sensors—may be based on optical images, geometric rays, interference fringes or any other optical feature or phenomenon described in the mathematics of geometric and physical optics.
It’s no secret that many of the analytical methods used by mechanical engineers are linearized simplifications of more or less nonlinear mathematical theories. Structural mechanics and heat transfer are just two of these theories. Much of the modern world is a product of these engineering simplifications. The region in which a linearized assumption is an adequate description is called a “small displacement domain.”
I have found this to be a great help in formulating optical figures of merit for inclusion in my engineering tools. For instance, in as much as the equations of physical and geometric optics are continuous in all of their derivatives, optics has small displacement domains in the same sense as structural mechanics: Over some small displacements, a linear assumption (based on the local first derivatives) is sufficiently accurate to predict changes in the optical performance metric of interest to acceptable precision. This is a mathematical certainty. The open questions are: “How big is the domain?” and “Is the inaccuracy acceptable?” These concerns are related: If the non-linearity is acceptably small, the small displacement domain is at least that large.
I have been applying the principles of overlapping small displacement domains (among structures, heat transfer and optics) in a finite element modeling tool that I call “Ebony” and that prepares “Optical Analog” finite element models. The models are analyzed in MSC/NASTRAN.*
Log in or become a member to view the full text of this article.
This article may be available for purchase via the search at Optica Publishing Group.
Optica Members get the full text of Optics & Photonics News, plus a variety of other member benefits.
